From a modern point of view and assuming that the fields were rectangular their areas have to be calculated by multiplying length and width. For this purpose, the measures of length and width would have to be converted into the same unit, preferably into the smallest unit nindan. The numbers of these units would have to be multiplied by some kind of algorithm, followed by a conversion into the standard area measures. But the evidence on the extant tablets precludes this interpretation.
The calculation of areas by multiplying the number of units of the length by the number of units of the width presupposes that the rectangular area is conceived as being covered with unit squares the number of which is defined as the size of the area. But the idea to determine the size of an area by covering it with squares was an unfamiliar idea to the whole Babylonian mathematical tradition. This is because the surveyors who coined the basic geometrical concepts created what Solomon Gandz described as a geometry of lines, that is, a geometry without any concept of an angle. The size of an area was conceived as being determined by the length and width of the area independent of its shape. Any area with equal length and width was considered to have the same size. This kind of geometry was perfectly adapted to carry out the task of the surveyors to determine the sizes of fields making use of only length measurements.
The ancient scribes used a calculation procedure based on the knowledge of the area for each pair of units of length and width measures:
- If the length equals 1 nindan and the width 1 nindan then the area equals 1 sar.
- If the length equals 1 nindan and the width 1 eš2 then the area equals 10 sar.
- If the length equals 1 nindan and the width 1 UŠ then the area equals 60 sar.
- If the length equals 1 eš2 and the width 1 eš2 then the area equals 1 iku.
- If the length equals 1 eš2 and the width 1 UŠ then the area equals 1 eše3.
- If the length equals 1 UŠ and the width 1 UŠ then the area equals 2 bur3.
The procedure did not presuppose any arithmetical techniques that are unattested by the extant sources. The precondition for effective application of such a procedure was, however, the knowledge of the sizes of unit areas for all combinations of length and width units. Tablets dating back to the first half of the third millennium, in fact, document that learning the sizes of such unit areas was an important part of the training of the scribes.
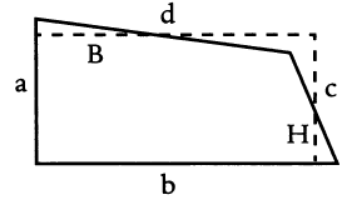
In order to solve the problem to calculate the size of such fields, the surveyors of the third millennium Mesopotamia already used what is called the surveyors’ formula. This formula is well-known, in particular from later Greek and Roman surveying technology. The formula determines the area of an irregularly shaped polygon with four edges (line forming the boundary) as the result of the multiplication of the means of opposite sides.
The surveyors’ formula is described in Rivista degli studi orientali, 2011, Nuova Serie, Vol. 84, Fasc. 1/4 (2011), pp. 11-16 with the name “field surveyors’ formula” as shown below:
The practice of field measurements is well attested in the corpus of the archaic texts. The administrative process involved three steps: measurement of the sides of a field in-situ (in its original place), calculation of the plot area by using the “field surveyors’ formula”, registration of the calculated sides of the equivalent rectangular plot.
With reference to the figure above, the formula is:
$A = B \cdot H = \frac{(b + d)}{2} \cdot \frac{(a + c)}{2}$
where B and H are respectively the semi-sums (i.e., the arithmetic means) of the two pairs of opposite sides. Consequently, the calculation of the area of the quadrilateral was reduced to the quite simpler calculation of the area of the (almost) equivalent rectangle.
The origin of the concept of multiplication is the following as per D. J. Struik, A concise history of mathematics, 4th rev. ed. New York: Dover Publications, 1987.
Number systems were accompanied by addition and subtraction operations to represent a count. For example, for the base ten number system, twelve was conceived as 10 + 2 or 9 as 10 - 1. This resulted in a primitive type of arithmetic. Multiplication began where 20 was expressed not as 10 + 10, but as 2 x 10.
To summarize, multiplication is repeated addition and the original concept of area (before the surveyors’ formula) was based on the knowledge of the area for each pair of units of length and width measures, like a rule and not like a mathematical function of length and width measures. But somehow the surveyors of the third millennium Mesopotamia started using the surveyors’ formula. And the original idea behind the use of multiplication (a method of counting) for area calculation is not given in the above-mentioned texts.
How did the surveyors’ formula originate, and how was the idea of using multiplication for area calculation conceptualized?