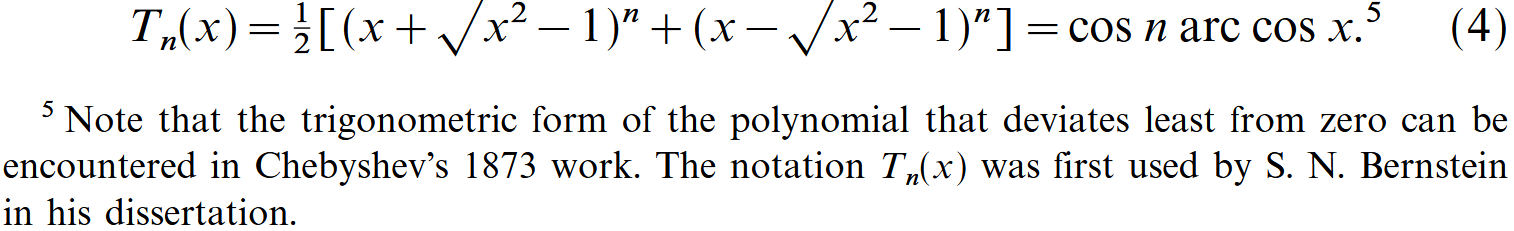According to wikipedia, Chebyshev polynomials were first presented in his paper Théorie des mécanismes connus sous le nom de parallélogrammes (1854), but the notations $T_n$ and $U_n$ don't seem to appear here. Who introduced and popularized this notation?
-
3$\begingroup$ No idea who introduced it nor who popularised it but presumably you do realise that his name was often transliterated starting with a T? If that is accepted then U follows T in the English collating sequence. $\endgroup$– mdeweyCommented Nov 27 at 16:52
-
1$\begingroup$ Decades ago in differential equations class we joked that the most difficult question to be asked was 'spell Chebyshev' given how many different ways various books spelled it. It even gets a section in en.wikipedia.org/wiki/Pafnuty_Chebyshev $\endgroup$– Jon CusterCommented Nov 27 at 17:12
-
2$\begingroup$ To follow up on @JonCuster 's remark, certain Russian sounds are translated variably from Cyrillic script into other languages. In German it is typical to translate Chebyshev as Tschebyschow or Tschebyscheff. An oft used French version is Tchebychev. I suspect the leading T in these transliterations is a decent lead as to why T became the normative letter associated with the Chebyshev polynomial of the 1st kind. Chebyshev himself published in French, and French and German were important languages of mathematics in the 19th century. $\endgroup$– Georg EsslCommented Nov 27 at 17:42
-
1$\begingroup$ There is a whole book where the main subject is how to spell Chebyshev's name: amazon.com/Thread-Mathematical-Yarn-DAVIS/dp/081763097X One of the funniest books I ever read. $\endgroup$– Alexandre EremenkoCommented Nov 28 at 14:04
1 Answer
- Goncharov, V. L. (2000). The theory of best approximation of functions. Journal of Approximation Theory, 106(1), 2-57.
Gonchorov discussing historically approximation theory contains the following footnote:
Indeed the notation can be found in Bernstein's 1913 dissertation (see page 52):
Conifold provided the following translation for the bottom paragraph via google translate:
For brevity we will in the following call c.$T_n(x)$, where c is a constant, trigonometric polynomials, and derive some of their properties, analogous to the property, discovered by Chebyshev.
Conifold hence rightly points out that for Bernstein "T" apparently is for trigonometric. Hence for Bernstein it was not a variant of Tchebychef that has since become an oft repeated explanation for the letter (compare Rivlin (1974) The Chebyshev Polynomials. p. 4. and Mason and Handscomb (2003) Chebyshev Polynomials. Appendix A). To be sure the current attribution is helpful in the context of orthogonal polynomial classification as they, in general, are attributed to a name (compare Abramowitz & Stegun (1965) p. 774f.).
It is unclear when exactly $U_n$ was introduced. It appears, together with $T_n$ in Pólya, G., & Szegö, G. (1925). Aufgaben und Lehrsätze aus der Analysis. Vol 2. Springer, p. 75. I do not know of an earlier source using this notation. I suspect that the notation was introduced into the English literature via Szegö (1939) Orthogonal Polynomials, AMS.
Incidentally the introduction of the Chebyshev polynomial of the second kind is credited to Korkin and Zolotarev (1873) by Steffens, K. G. (2006) The history of approximation theory: from Euler to Bernstein, Birkhäuser, p. 92.
-
1$\begingroup$ @conifold I have added a screen capture from the thesis to my answer. It's on the very first page after preface text. Page number is 52. $\endgroup$ Commented Nov 28 at 10:29
-
4$\begingroup$ Google translates the sentence right under as "For brevity we will in the following call $c.T_n(x)$, where $c$ is a constant, trigonometric polynomials, and derive some of their properties, analogous to the property, discovered by Chebyshev." So "T" apparently is for trigonometric. Ironically, Bernstein does not use "$T_n(x)$" in the following. $\endgroup$– ConifoldCommented Nov 28 at 11:06
-
2$\begingroup$ @conifold Incidentally I'm doing more digging, and it may well be that the innovation in Bernstein is the (x) functional notation. For example in both Chebyshev (1874) and Kirchberger (1902) one finds T_n without (x). $\endgroup$ Commented Nov 28 at 11:18
-
1
-
3