Well, I am not sure if this is the right place to ask but here it goes (if this forum is not the right place to ask, I apologize and certainly moderators will delete my question): i am very curious about the authorship and history of this calendar design(the clever compact one page calendar: https://medium.com/starts-with-a-bang/this-one-page-calendar-will-change-how-you-view-the-year-b8ecad85eebd
as a gift here goes the 2024 one page calendar:
The web-page linked by this question ("Who invented ... ?") credits the 'one-page calendar' design (for a year) to "E Siegel" -- along with a phrase that suggests he is also claiming a copyright. (It's not necessary for this answer to go into the degree of originality in the design, or whether it amounts to an invention, etc.)
There are many possible ways to write out a calendar -- using 'calendar' here to mean, as in the example given, an arrangement of tables or lists that tells, for any date defined by month and date-number within a given year, on which weekday it falls.
Most or all of such lists effectively use a part of the information originally given long ago in the papers that defined the Gregorian calendar reform of 1582, and/or in the wide variety of listings in prayer-books and other subsequent works to define the date of Easter. The two main functions contained in these accounts were (i) to define how the days of the week match up with the calendar dates in the year, and (ii) also how the new and full moons match up with the dates in the year.
Calendars such as those in the present question need only part (i) of the data just mentioned. The information in that part is readily compiled by recognizing that there are (a) just seven arrangements of the monthly calendar, where the 1st of the month falls on each one of the seven weekdays (allowing variability of month-length from 28 to 31 days according to the case); and (b) just seven pairs of annual calendars, each defined by the weekday of January 1, and within each pair, whether the particular year is a common or leap year.
The original matching, for years of the modern (Gregorian) calendar, between the days of the week and the numerical month-dates, is found in "Canon 4 : De litera Dominicali" from the implementing documents accompanying or following the 1582 papal bull "Inter gravissimas ...". For present and practical purposes, the necessary information can also be found, summarised much more briefly and usably, for example at page 427 in the 1961 "Explanatory Supplement to the Astronomical Ephemeris ...". (Both of these cited works define the weekday-year relationship by the 'Sunday letter', which is 'A' where the first Sunday of the year is on January 1; 'B' where it is on January 2; and so on up to 'G'.)
Since the question asks about invention, authorship and history, it is relevant to go back to the Latin original of Canon 4, which can be found reproduced in a book of 1603, "Romani Calendarii A Gregorio XIII. P.M. Restituti Explicatio" by Christopher Clavius, who was a leading technical member of the papal calendar commission that accepted (and modified) the calendar reform proposal of Aloysius Lilius. (Lilius is sometimes credited with proposing the entire calendar reform, but it can be seen from the 1577 consultative booklet containing his proposal "Compendium novae rationis restituendi calendarium ...", as well as from the references to it in the text of 'Inter gravissimas..,' that its concern is to deal with the (more difficult) issues about Easter full moons and related matters; it does not originate the basic reform of the solar part of the calendar, for which the proposal was improved from ideas of older origin.) Clavius may therefore be the effective designer/author/compiler of the information in Canon 4 as presented (leaving aside the imponderable question whether it was also ever something 'invented').
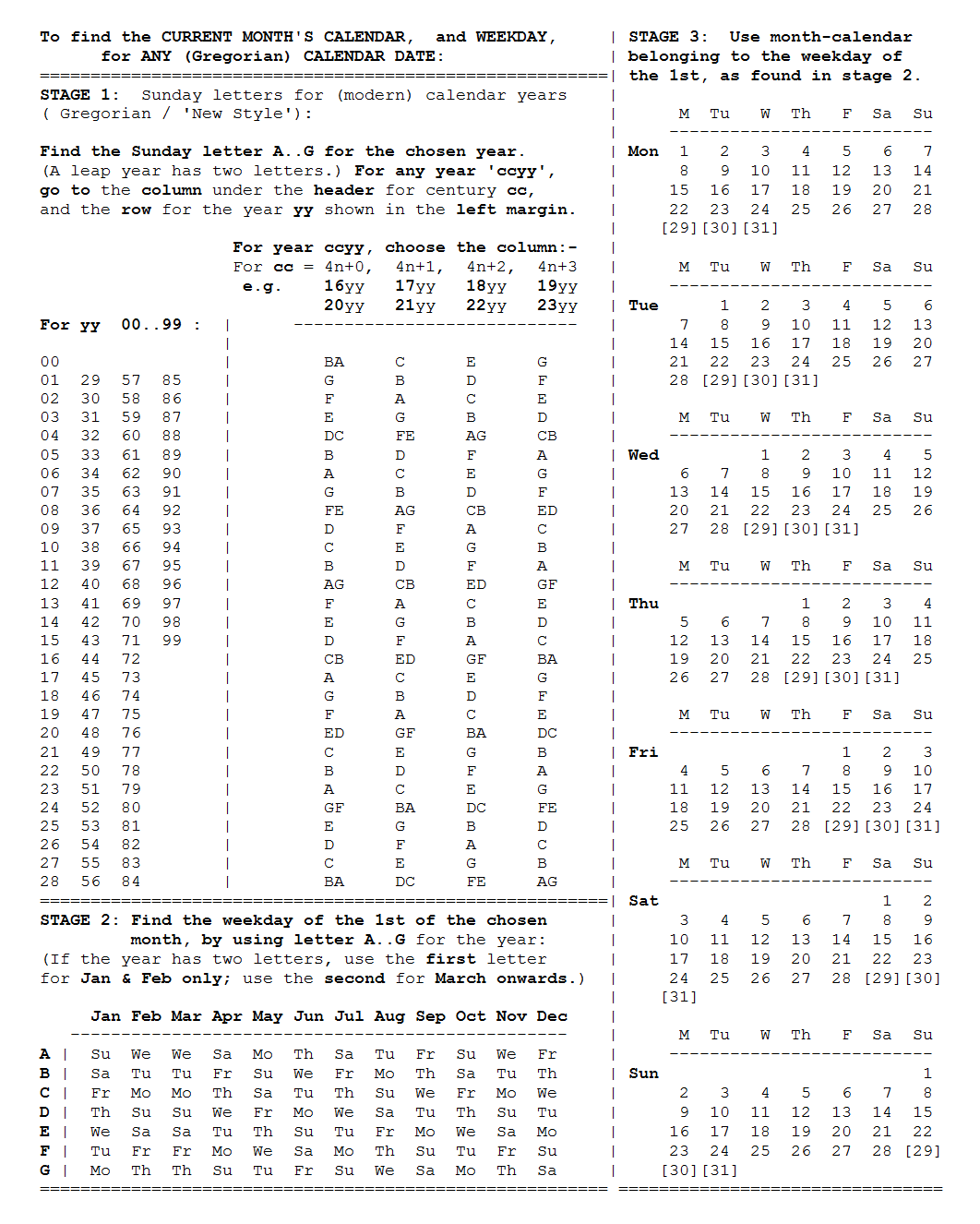
So far, this deals with the information content of the 1-page calendar. If information is desired about the origin of details of the layout, this seems very difficult to find. But it seems relevant to notice that while the illustrative 1-page calendar for 2024 presented in this question does effectively compress a year's calendar into a single table, there are also many other other possible ways of presenting the same and more information about calendar days and dates. For example, the accompanying page-image (below) illustrates a way of fitting, into a single A4 page (with instructions for use), the day-date information not only for one year, but also for any and all years of the modern (Gregorian) calendar. Readily derivable from this is the information contained not only in the the 1-page calendar example for 2024 given in the question, but also in the corresponding calendar for any other year. (The attached page image was compiled by the present poster, but there is no wish to claim copyright.)
