3
$\begingroup$

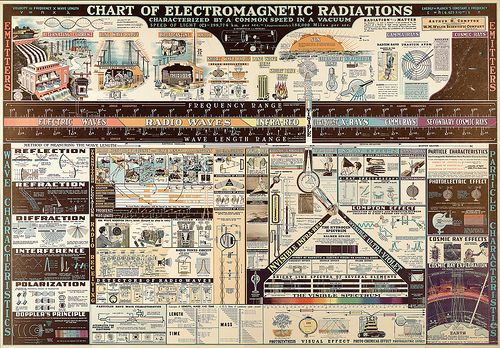
The meter and second, at least, have been redefined several times since they became common in science. Sometimes when referencing historical documents, it's useful to know how the units of the time relate to units in the modern day, but I'm finding it hard to locate that information by web searches. Does anyone know a good source (or can provide the information directly)?

For example, when referencing this chart (shared by Livermore National Lab),

test

I'd want to know what the length of a 1944 meter is in current day meters, and similarly for the second, to check whether they account for the difference in the reported values of the physical constants $c$ and $h$.

Improve this question
$\endgroup$
6
  • 1
    $\begingroup$ The history of definitions of the meter is clearly explained in Wikipedia en.wikipedia.org/wiki/Metre. $\endgroup$
    – Alexandre Eremenko
    Commented Dec 7, 2015 at 22:48
  • 1
    $\begingroup$ @AlexandreEremenko yes, but that doesn't help me as I'm asking for values, not a qualitative history. That information is not in Wikipedia. $\endgroup$
    – David Z
    Commented Dec 8, 2015 at 4:41
  • $\begingroup$ The Wikipedia article gives you values. $\endgroup$
    – Alexandre Eremenko
    Commented Dec 8, 2015 at 13:50
  • $\begingroup$ @AlexandreEremenko where? Can you point me to the part of the article that lists values? $\endgroup$
    – David Z
    Commented Dec 9, 2015 at 4:26
  • 1
    $\begingroup$ @VicAche Yes, I looked at that table. That's not what I'm looking for. It gives uncertainties and qualitative definitions, not values. $\endgroup$
    – David Z
    Commented Dec 9, 2015 at 14:26

1 Answer 1

Reset to default
2
$\begingroup$

Sometimes when referencing historical documents, it's useful to know how the units of the time relate to units in the modern day, but I'm finding it hard to locate that information by web searches.

You can't find that information because it doesn't exist; the values haven't changed. The various redefinitions of the meter did not change what a meter represents. They instead made the definition more precise. The current definition of the meter is consistent with the prototype metre bar constructed in 1889. Maintaining consistency across definitions is a key precept in metrology.

Violating this concept is something that should be done with great care and great fanfare. This happened with the liter, which was redefined in 1964 to be exactly a cubic decimeter (or 1/1000 of a cubic meter). This was inconsistent with the pre-1964 liter, which was based on the volume occupied by a kilogram of pure water at 4 °C. Per this prior definition, a liter was a bit larger than a cubic decimeter, by about 28 parts per million. The inconsistent redefinition in 1964 was only made after significant debate and consensus, and it received a lot of fanfare.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Well, I know there has to be consistency between definitions, but the definitions have some associated uncertainty. Consistency would imply that the new definition should fall within the uncertainty of the old definition, but the new mean of the probability distribution won't be the same as the old one, in general - or such was my understanding, at least. What I'm looking for is basically the ratios of old to new mean values. $\endgroup$
    – David Z
    Commented Dec 9, 2015 at 4:25
  • 1
    $\begingroup$ @DavidZ we pointed at those uncertainty. "Not changing the value" means not changing the mean value, not defining a new value that lies within the uncertainty. If you can't understand this then your question is no more about the history of science but about your understanding of science itself. $\endgroup$
    – VicAche
    Commented Dec 9, 2015 at 17:59

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.