I am not sure if this is exactly the statement of Faraday's paradox, but it is similar enough for my question.
In the late 19th century Faraday's law of electromagnetic induction was often expressed as something like "The emf per unit length in a circuit is proportional to the rate at which this unit length cuts or is cut by lines of magnetic induction". Here the emf per unit length would be the total force per unit charge $\vec E+\vec v×\vec B$ and the lines of magnetic induction represent our $\vec B$ field.
This accounts for the emf due to the motion of a wire, as the wire cuts through the lines of magnetic induction; the emf due to the magnet moving relative to the wire, as the lines move together with the magnet and cut through the wire; and several cases of emf on a secondary due to variation of currents in a primary, as the current in the primary varies the lines of magnetic induction expand and contract toward and away from the primary wires and cut through the wires in the secondary. This does miss cases where there is an emf without a magnetic field present, such as a secondary wound around a toroidal transformer, but these are not important for my question (and have somewhat been dealt with by some authors, I believe)
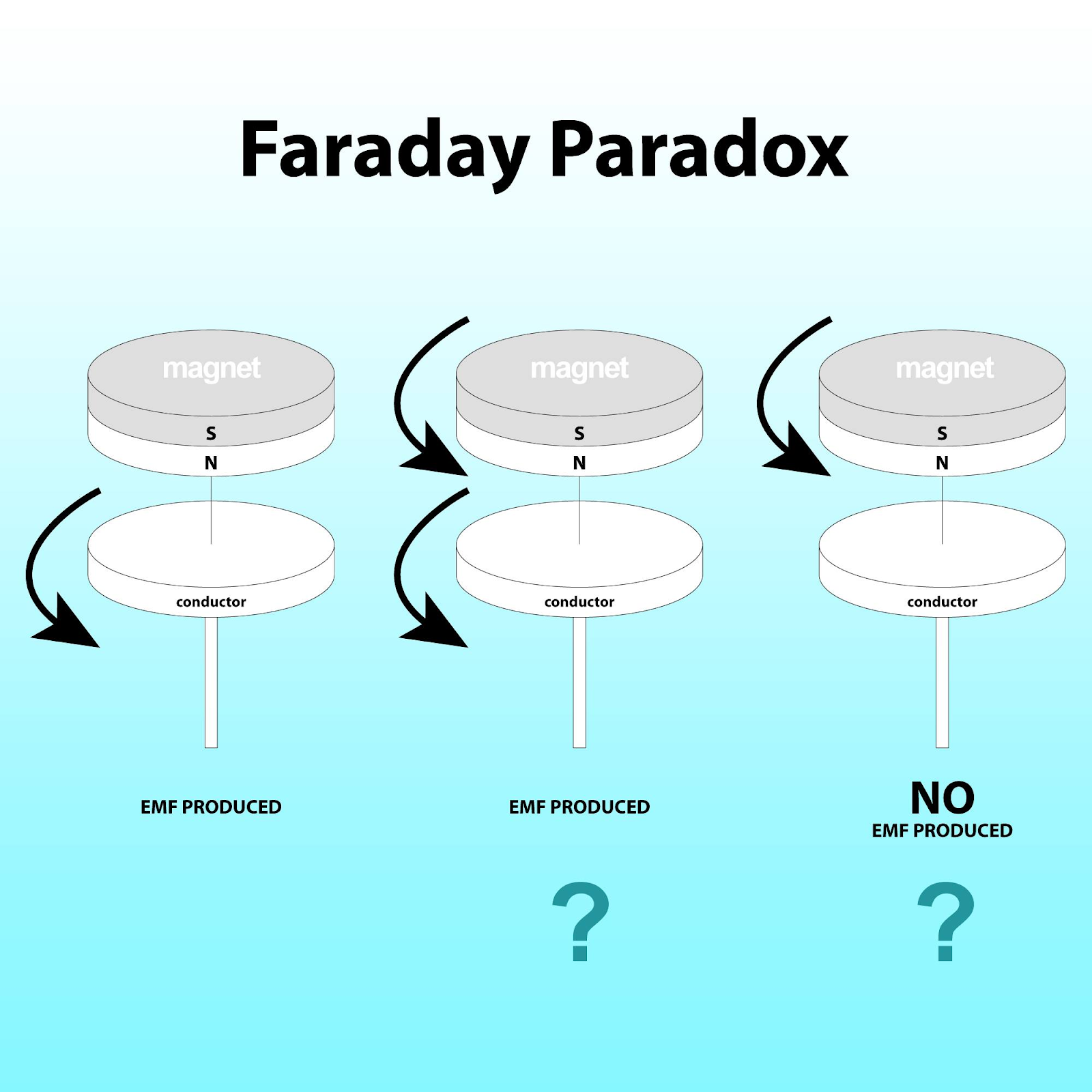
In a Faraday generator such as the one pictured, a conductive disk is placed together with a magnet (in this diagram a symmetric disk magnet). It is found that when only the conductive disk is spun, or both disks are spun together an emf is generated between the disk's axis and its edge. However, when only the magnet is spun there is no induced emf.
In our modern view, this is easy to explain. When the disk moves its electrons are moving in a magnetic field so they experience a force. The magnetic field is a property of space that exists independently from the magnet. The only way for the magnet to induce an emf in the disk when it is still would be to produce an electric field through a changing magnetic field, and the magnet is symmetrical so its rotation doesn't change the magnetic field in any way.
But back then, many physicists considered these lines to be somewhat physical and intrinsically linked to electric charges (or circuits of electric currents). In fact, J. J. Thomson developed a theory of electric field lines where the lines move together with the electric current and this motion produces magnetic fields. These lines begin and end on electric charges, and are 'pulled along' as they move. Under this view, when a charged disk spins it 'drags' the electric field lines along with it, producing a magnetic field. This is observed, of course, it is called a convection current.
On the other hand, in the absence of magnetic charge, the magnetic field lines don't begin or end, but instead loop back into themselves. Instead, they are linked with lines of electric current (or displacement current), as per the Ampère-Maxwell equation. In a magnet, the currents from adjacent amperian loops cancel out, so the magnetic field lines are unable to link with the individual loops and move along with them. There are only able to link with the total bound current which flows around the magnet, so they will not be moved by a rotation of the magnet (except for maybe an increase in intensity, as the rotation increases this 'flow of current')
That is basically my line of reasoning, as it recently occurred to me. Have any physicists ever put forward a similar argument?