Isaac Newton is known as the discoverer of the FTC (Fundamental Theorem of Calculus), so maybe he wrote the integral symbol and derivative symbol. I know he wrote the derivative symbol as $\dot y$ but I cannot find the integral symbol he wrote. How did Isaac Newton write the integral symbol?
$\begingroup$
$\endgroup$
3
-
1$\begingroup$ And see en.wikipedia.org/wiki/Integral#Historical_notation $\endgroup$– peter a gCommented Mar 30, 2023 at 15:14
-
1$\begingroup$ As far as I think by Wikipedia, Newton's notation is like $\fbox{$x$}=\frac12x^2+\mathit{Const.}$. $\endgroup$– Username_qsCommented Mar 30, 2023 at 15:32
-
$\begingroup$ @MIKANkankitsu I would like to know the answer to this also. The tall S $\int$ is agreed to have been invented by Leibniz, so Newton almost certainly didn't use it. $\endgroup$– Mark DominusCommented Mar 30, 2023 at 15:48
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
1
Newton used both vertical bars ($\overset{|}{x}$) and rectangles ($\boxed{x}$) to denote integrals in his Quadratura curvarum published in 1704.
Here, the bar notation is used on the bottom of page 9 in the linked digital scan (multiple bars would represent multiple integrals):
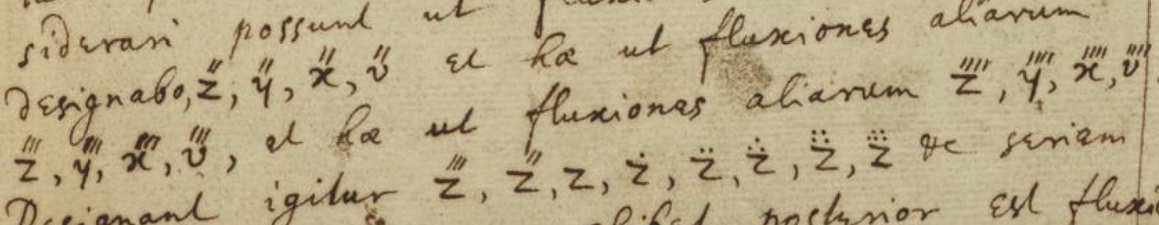
This source states that the notation did not become popular because the bar could be misinterpreted as a "prime", and a rectangle was difficult to print at the time.
Additional information from post linked by @KurtG. in the comments:
- Newton used $\overset{|}{x}$ to indicate the quantity whos fluxion (a fluent was a changing value and a fluxion was its instantaneous rate of change) is $x$.
In fact, it seems this Wikipedia article sums everything up very clearly. Given $y=f(t)$, $$ \overset{|}{y}=\square y=\boxed{y}=\int y\ \mathrm{d}t. $$
-
1
$\begingroup$
$\endgroup$
The section II.4 Integral Calculus in Analysis by Its History by E. Hairer and E. Wanner begins with quotations, one of them from a letter by Newton:
Newton, letter to Keill, April 20, 1714: And whereas $\mathrm{M^r}$ Leibnits praefixes the letter $\int$ to the Ordinate of a curve to denote the Summ of the Ordinates or area of the Curve. I did some years before represent the same thing by inscribing the Ordinate in a square ...
My symbols therefore ... are the oldest in the kind.
